Corps de l’article

C. S. Peirce (1839-1914) had a keen interest in mathematics, semiotics, and the most mathematical economics of his era. Peirce’s interest in semiotics is somewhat well-known in certain lines of scholarship, but his semiotic approach to mathematics and his interests in mathematical economics are much less well-known. The goal here is to bring Peirce’s interests in semiotics, a semiotic approach to mathematics, and his interests in mathematical economics together for what may be the very first time. Peirce read and wrote about the mathematical contributions of prominent economists in the history of economics such Cournot and Jevons and he at least mentions Ricardo, Marshall, and Walras. Stemming from those interests, Peirce did provide a mathematical, optimizing model of the insurance firm as his most elaborate example of pragmatism in the Harvard Lectures of 1903. These instances of mathematical economics in Peirce’s writings are of even broader significance. Peirce chose economic examples to illustrate the more semiotic and mathematical aspects of his philosophy of pragmatism. With regard to semiotics, a conception of diagrams plays a central role. Peirce’s very broad interpretation of “diagrams” includes not only geometric and logical graphs but just about any ordered array of letters, numbers, symbols, and words. The creativity of Peirce’s philosophy of pragmatism has been recognized for at least a century. However, its deep mathematical nature, but for a few exceptions, is mostly of more recent vintage. This more mathematical and logical version of pragmatism is what Peirce (1905a, 1905b) preferred to call “pragmaticism”. In one of his more publicly acclaimed lecture series, the Cambridge Conference Lectures, Peirce (1898 : 267) actually referred to this outlook as his mathematical cosmology.[1]

More than a century later, Peirce’s evolutionary philosophy became prominent in another significant work on economics. Just a few years ago, Carsten Herrmann-Pillath (2013) authored a long treatise on evolutionary economics with semiotics as a central aspect of that work. Among several significant influences like Veblen, Hegel, and Georgesu-Roegen, Herrmann-Pillath quite explicitly adopted important aspects of the evolutionary philosophy of Peirce and especially his conception of semiotics. Herrmann-Pillath’s aim was to construct a broad natural philosophy of economics such as a 19th century intellectual figure such as Hegel might have done. Herrmann-Pillath in important ways does make extensive use of applied mathematics. He even raises matters of abstract mathematics as they relate to understanding the function of the human mind in evolutionary processes. In the treatise, significant use is made of equations from several scientific disciplines and there are references to abstract mathematics, but mathematics per se is not given a semiotic interpretation. Herrmann-Pillath’s work does make extensive use of diagrams to explain broad patterns of economic activity with the most important ones elaborating Peirce’s semiotics. Since diagrams are a central feature of Herrmann-Pillath’s work and likewise in Peirce’s semiotic philosophy of mathematics, the treatise thus has something of a Peircean, semiotic, mathematical character. These similarities and differences between Peirce and Herrmann-Pillath on semiotics, mathematics, and economics are of intrinsic interest.

The Multi-Stranded, Somewhat Topological Character of Peirce’s Writings and Manuscripts

There are several somewhat partially overlapping interpretations of Peirce and his pragmatism. Perhaps most prevalent is that of the pragmatist-philosopher with other, somewhat separable secondary interests including logic, science, scientific method, mathematics, semiotics, and a sliver of economics. Often the secondary interests fade into the background in the literature about Peirce. Philosophical primacy in interpreting Peirce is quite understandable. There is a great stretch of Peirce’s writings which are predominantly philosophical with many articles and lecture series that are either predominantly philosophical or include a significant philosophical essay or two. Additionally there are several longer, monograph-length manuscripts, which are predominantly philosophical. The predominant intellectual image of Peirce as a philosopher was reinforced by the first large collection of his writings, the eight-volume (in 4) Collected Papers (CP). Most of the volumes contain philosophical writings, but for volumes three and four which reveal selections of mathematical and logical contributions. Several decades ago, a chronological edition of his works began to appear with editorial work in Indianapolis and published by Indiana University Press. Now we have seven volumes of The Writings of C. S. Peirce : A Chronological Edition (W, 1982-2010) and the companion, two-volume introductory collection, The Essential Peirce (EP 1-2, 1992, 1998). In this collection, one can see how any of Peirce’s writings appear in the context of others. Manuscript versions of previously published writings appear as well. Additionally there are smaller collections such as Chance, Love, and Logic (1923) edited by Morris Cohen, whose title refers to Peirce’s three fundamental modes of evolutionary processes : evolution by chance, by higher purposes, and by mechanical necessity.

While Peirce was at Johns Hopkins University in the early 1880s, he wrote several dozen mathematical papers and over a period of 15 years or so, several major scientific reports were written. In 1976 Carolyn Eisele published the large four-volume collection of Peirce’s writings on mathematics titled, New Elements of Mathematics (NEM) and about a decade later in 1985 the two-volume Historical Perspectives on Peirce’s Logic of Science : A History of Science (HP). By itself the New Elements appear to equal if not surpass the published contributions in the Collected Papers and the Historical Perspectives provide a large window on Peirce’s scientific contributions. One also encounters a great deal more on Peirce’s conception of semiotics and his semiotic interpretation of mathematics in these volumes. Furthermore, there is a tiny sliver of writings on economics which are of significant interest as well.

The New Elements (NEM) and Historical Perspectives (HP) volumes provide the opportunity to consider the notion that mathematics is central to Peirce’s philosophical vision. One might hypothesize that these volumes are equal in importance to other strands of his writings. Thus, these volumes lead to an entirely different sense of Peirce’s lifetime contributions. Peirce has a very different interpretative style than what prevails in much of Western thought and science. His broadest ideas are continually intertwined with detailed depictions, explorations, and results from other disciplines. This creates something like an intellectual labyrinth where no discipline or interpretative framework ever stands alone. Of even more significance is the elevation of the relative importance of mathematical writings including semiotics.

An even less recognized mathematical aspect of Peirce’s outlook and writings is their somewhat topological character, not just in terms of subject matter, but also in terms of the patterns of the strands of arguments, content, and style of discourse. The metaphors of strands, labyrinths, and interconnected networks of interrelated writings clearly have their counterparts in topology. Topology is a field of mathematics, which as Peirce came to know it, is concerned with the abstract properties of lines, curves, surfaces, spaces, solids, and knots. Whatever patterns one encounters in any domain can be assigned representation with letters, symbols, numbers, diagrams, and words. Peirce even wrote extensively about topology (1895a, 1895b).

What is perhaps even more important is that Peirce’s intellectual process itself became much more topological as he wrote his monographs, articles, manuscripts, and scientific reports. Every writing has this topological character. Every strand is interconnected with every other strand even when two or three dominate in any monograph or writing. Since mathematical and philosophical strands are always present at their most abstract levels, this can make any particular writing or monograph particularly daunting. Yet even when Peirce descended into the smallest details in whatever was the main subject of the moment, the next paragraph could leap to the opposite end of the spectrum to another strand at the highest levels of generalization. During the last two decades of his active intellectual life, Peirce wrote what amounts to a constellation of multiple intellectually, mathematically, and scientifically interrelated monographs and he gave several public lecture series, which are also of monograph-length. They all have this multi-stranded-labyrinth, topological-like intellectual character.

Peirce’s Writings on Semiotics

Beyond the main strands of philosophy, logic, mathematics, and the nature or human inquiry and inference, within the labyrinth of Peirce’s writings and ideas there are other lines of interest such as semiotics and economics. In comparison to philosophy and mathematics, there is much less on semiotics and even less on economics. Peirce first wrote about semiotics in the late 1860s when he was in his late twenties and then more in the last two decades of his life. Perhaps this is because during the middle of his career, Peirce conducted gravity measurements for the U. S. Coast Survey and for five years lectured at Johns Hopkins University from 1879 to 1884. He returned to semiotic themes during the last creative stretch of his life -- the last decade of the 19th century and the first decade of the twentieth century. The writings that stand out with regard to semiotics are the Lowell Lectures (1903d).

Comments by Peirce on what would become a theory of signs appear in several early writings. In the late 1860s, before he would turn 30 years of age, several prominent passages on semiotics can be found in three different published articles. “In a “New List of Categories,” in the context of writing about philosophical categories, Peirce (1867) asserted that there are three kinds of representation : likenesses, indices, and general signs which may also be called symbols. In another writing published the next year, these three forms of representation seem to take on much broader significance. In “Questions Concerning Certain Faculties Claimed for Man,” Peirce (1868a), Peirce wrote :

From the proposition that every thought is a sign, it follows that every thought must address itself to some other, must determine some other, since that is the essence of a sign….To say, therefore, that thought cannot happen in an instant, but requires time, is but another way of saying that every thought must be interpreted in another, or that all thought is in signs.

1868a : 24

In yet a third article, Peirce further commented on important aspects of signs and their function in cognition. At one point he asserted, “We have no power of thinking without signs” (Peirce 1868b : 31).[2] Thinking in signs is related to drawing inferences since the triadic sign process always involves an inference. Inference requires a special arrangement of signs known as the syllogism. With regard to the inner processing of ideas as signs, he remarks, “Something, therefore, takes place within the organism which is the equivalent of the syllogistic process” (1868b : 31).[2] At a later point, he returns to the theme of representation and claims that it occurs in consciousness as a sign. A sign has three roles : “Now a sign has, as such, three references : 1st, it is a sign to some thought which interprets it; 2d, it is a sign for some object to which in that thought it is equivalent; 3d, it is a sign, in some respect or quality, which brings it into connection with its object,” (Peirce 1868b : 38). A significant property of a sign is that it has a real, physical connection with its object (ibid. : 38).

Nearly a quarter of a century later, Peirce again took up his ideas on semiotics. One of his writings, “What is a Sign?”(1894), was intended as part of a monograph. In that piece, Peirce began by considering an individual in a dream-like state who is aroused by a loud and prolonged whistle. Here Peirce was concerned to explore what it means to think about the sound of the whistle. What could such a loud sound mean? Then he launches into an account of his theory of signs maintaining that there are likenesses or icons, that there are indications or indices, and that there are symbols or general signs. A year later another account of his theory of signs is found in “Of Reasoning in General” (Peirce 1895c : 13-18).

Peirce’s most detailed writings on semiotics appear in the Lowell Lectures of 1903. Earlier in that year, Peirce (1903a) had delivered his “Lectures on Pragmatism” at Harvard. In those lectures, Peirce had begun by reframing his conception of pragmatism in Lecture I with his most prominent illustration of the logic of pragmatism from mathematical economics and the optimizing calculus of the insurance firm (Wible 2014). Then he took up matters about how humanly experienced events appear to the mind and he created his own version of phenomenology. Those lectures end with a return to logical themes related to the process of abduction.

For the Lowell Lectures, Peirce (1903e) prepared a “Syllabus” in advance of the lectures. It is selections from the “Syllabus”, which contain Peirce’s most developed writing on semiotics. In one section is found “An Outline Classification of the Sciences” (1903e : 252-262). There semiotics was located as being part of logic. This classification of the sciences places those sciences which are most abstract at the highest level and then proceeds to other sciences which make use of those which are more abstract. The first level of classification are sciences of discovery, sciences of review, and practical sciences. The sciences of discovery are mathematics, philosophy, and applied sciences. Mathematics is at the top of Peirce’s classification of the sciences and considered to be more abstract than philosophy. Philosophy comes next and is further divided into phenomenology, the normative sciences, and metaphysics. Then the then normative sciences are subdivided into three branches : esthetics, ethics, and logic. It is logic that deals with signs and especially one of its most prominent sub-branches, speculative grammar. In this regard, Peirce writes : "All thought being performed by means of signs, Logic may be regarded as the science of the general laws of signs. It has three branches : (1) Speculative Grammar, or the general theory of the nature and meanings of signs, whether they be icons, indices, or symbols…." (Peirce 1903e : 260) The other two branches of logic are Critic, which deals with classifying arguments and Methodeutic, which studies the methods in the investigation of truth.[3]

While one could continue towards a more detailed exposition of Peirce’s semiotics, here we are more interested in an overall sense of the significance of his theory of signs. Fortunately, we have what can be regarded as a succinct and highly informative overview of Peirce’s semiotics and philosophy. Nathan Houser, a former long-time editor of the Writings of Peirce, comments on another philosopher who subsequently authored one of the most comprehensive works on Peirce’s theory of signs, Thomas Short. Referring to his work, Houser summarizes how semiotics fits within the overall landscape of Peirce’s ideas[4] :

According to Thomas Short, on the other hand, Peirce is a semiotic realist. The decision to label Peirce one way or the other seems to reflect the relative importance one attaches to the different elements of the sign relation, and often seems to be a matter of emphasis rather than a divergence of doctrine. Since he explicitly embraced a more and more encompassing realism, it might seem more appropriate to follow Short and call Peirce a semiotic realist – especially as that reflects his pragmatic admonition that our conceptions are meaningless unless they have reference to something outside of intellect

Houser 1992 : xxxv

Then, from this more general conception of semiotic realism, Houser offers a brief summation : “A sign is anything which stands for something to something. What the sign stands for is its object, what it stands to is the interpretant. The sign relation is fundamentally triadic; eliminate either the object or the interpretant and you annihilate the sign” (ibid. xxxvi).

Peirce on Mathematics and Semiotics

Semiotics is one of those strands of the labyrinth of Peirce’s writings that has received less attention than the main philosophical narrative especially among economists. As just outlined, there are writings where Peirce makes semiotics a crucial central aspect of his conception of inquiry and meaning. Something similar is true with his semiotic interpretation of mathematics. Certainly, there are a significant number of writings about mathematics and a first impression is that mostly they form an entirely separate line of interest for Peirce. This creative philosopher was also an innovative mathematician. But that would miss the major role that mathematics plays in Peirce’s classification of the sciences. It would also miss his semiotic interpretation of mathematics, which has been rarely discussed by those emphasizing the semiotic interpretation of Peirce with the exception of someone like Fisch (1978). After an explanation of Peirce’s semiotic interpretation of mathematics, one can see how it extends and adds to his overall interpretation of the role of signs in human inquiry and even includes a computational dimension. For whatever reason, most treatments of Peirce’s philosophy of signs rarely if ever extend a conception of semiotics beyond applied mathematical science to computation. But Peirce did. There is a significant sense in which Peirce’s semiotic conception of applied mathematical science including computation seems to be quite consonant with the research practices of many data and software-rich sciences early in the 21st century including economics.

In Peirce’s early writings about semiotics there are a few suggestive passages directly connected with mathematics. Before Peirce’s writings about signs in those three articles in 1867 and 1868, there are also drafts of manuscripts and a set of Harvard lectures where it appears that Peirce (1865a, W 1 : 174-175, 256-258, 282) is beginning to explore his conception of signs. In one of those, he briefly makes a comment about geometry and algebra (1865a : 306). That same year it is clear that, the intention was to create an “unpsychological” view of logic (1865b).

Less than a decade later, from a manuscript titled, “Of Logic as a Study of Signs,” he begins by proposing that “A sign is something which stands for another thing to a mind” (1872-73 : 82).[5] Then he describes three aspects of signs and how a sign addresses itself to the mind. Here he maintains that mathematics is a discipline where aspects of human mental processes can be investigated without psychology because of their intricate logical operations with signs :

The business of Algebra in its most general signification is to exhibit the manner of tracing the consequences of supposing that certain signs are subject to certain laws. And it is therefore to be regarded as a part of Logic. Algebraic symbols have been made use of by all logicians from the time of Aristotle, and probably earlier.

1872-1873 : 83-84

Another reference to mathematics in terms of a theory of signs comes more than a decade later in an 1885 article, “On the Algebra of Logic…..” The first section is titled “Three Kinds of Signs”. It begins by considering the character or nature of propositions. Then, by the second paragraph, the discussion moves to the subject of representing propositions by signs. He describes a sign as being “in a conjoint relation to the thing denoted and to the mind” (1885 : 162). As long as it retains its triple relationship, the sign remains abstract and general and he calls them “tokens”. Years later, he would just call this general type of sign, a symbol. A second form of signs can exhibit a dual relationship and is usually associated with natural and physical attributes, which he calls an index. A third type of sign depends on a non-physical relationship of resemblance, which he terms an icon. The iconic connections which are essential for the successes of mathematics comes through diagrams :

Icons are so completely substituted for their objects as hardly to be distinguished from them. Such are the diagrams of geometry. A diagram, indeed, so far as it has a general signification, is not a pure icon, but in the middle part of our reasonings we forget that abstractness in great measure, and the diagram is for us the very thing. So in contemplating a painting ….the distinction of the real and the copy disappears.

1885 : 163

In the next few paragraphs, Peirce goes on to argue that logic makes great use of tokens (symbols) and indices. He also claims that the relationships which connect signs and tokens can be portrayed with diagrams. Such diagrams are icons which facilitate reasoning about those relationships. This relational and semiotic understanding of logic can also help explain why mathematics is so powerful in helping us understand the world : “It has long been a puzzle how it could be that, on the one hand, mathematics is purely deductive in its nature, and draws its conclusions apodictically, while on the other hand, it presents as rich and apparently unending a series of surprising discoveries as any observational science.” (1885 : 164). Peirce goes on to claim that intricate relational reasoning with a diagram is a significant part of understanding why mathematics has been so successful :

The truth, however, appears to be that all deductive reasoning, even simple syllogism, involves an element of observation; namely, deduction consists in constructing an icon or diagram the relations of whose parts shall present a complete analogy with those of the parts of the object of reasoning, of experimenting upon this image in the imagination, and of observing the result so as to discover the unnoticed and hidden relations among the parts

1885 : 164

From mathematics, Peirce next goes to the most common form of the syllogism and asserts that the syllogism “is really a diagram of the relations” of its component propositions (ibid. : 165).

Throughout the passages on semiotics, Peirce often wrote at the level of the individual in an evolutionary process. The sense is that he wanted to explore the logic of how an individual is able to learn in an uncertain world. The process of evolution could shape how humans learned to construct and interpret abstract ideas that become formed as signs. A source of this shaping of abstract ideas was attributed to Galileo and his idea of il lume naturale (1891 : 287). An interpretation of this principle can be found in one of his original essays on pragmatism, “Order of Nature” :

It seems incontestable, therefore, that the mind of man is strongly adapted to the comprehension of the world; at least, so far as this goes, that certain conceptions, highly important for such a comprehension, naturally arise in his mind; and, without such a tendency, the mind could never have had any development at all.

Peirce 1878a : 318

This shaping of the human mind to understand and provisionally interpret how the world interfaces with the individual begins at a young age. It is also intertwined with that contingent reasoning process abduction. Abduction is another but more expansive concept for making hypothetical inferences about our external world. An abduction often is a hypothetical explanation conceived after the experience or observation of an anomalous surprise. In an early writing, the reasoning process that would later become abduction appears more simply in “Deduction, Induction, and Hypothesis” (1878b). In his later writings, such as the Harvard and Lowell Lectures of 1903, abduction took on a major role in his conception of how humans could ever come to understand their world. An abduction is a semiotically facilitated but contingent conjecture, which is provisionally adopted as an explanation that might account for that aforementioned surprising event. This process begins in a primitive way in the mind and experience of the young child :

Thus we have in order of strength, Deduction, Induction, Hypothesis [abduction] ....Yet it is hypotheses with which we must start; the baby when he lies turning his fingers before his eyes is making a hypothesis as to the connection of what he sees and what he feels. Hypotheses give us facts. Induction extends our knowledge. Deduction makes it distinct.

Peirce 1865a : 283

At a much later point in that individual’s human development, not only would the young human mind begin to symbolize what it sees and feels, it could acquire the capacity to conceptualize those connections as a logical relation. As found in the second Harvard Lecture, Peirce (1903a : 155) would claim that humans have the possibility of “cognizing a relation”. In a draft of one of those first articles with significant passages on semiotics, Peirce (1868c : 173) would claim, “All thought, therefore must necessarily be in signs, […] From this proposition that thought is a sign it follows that every thought must address itself to some other, must determine some other, since that is the essence of a sign.” At a later point, he would more generally assert, “Logic itself is a study of signs” (Peirce 1898 : 146).

From logic and human thought in more general terms, one can turn again to mathematics. Moving beyond the depiction of abstract semiotic thought beginning with the young child, Peirce would baldly claim, “All things, forms, symbols are symbolizable” (Peirce 1865a : 282). In “What is a Sign”, Peirce likens the reasoning of mathematicians to other interpretative processes that depend on likeness : “The reasoning of mathematicians will be found to turn chiefly upon the use of likenesses, which are the very hinges of the gates of their science. The utility of likenesses to mathematicians consists in their suggesting, in a very precise way, new aspects of supposed states of things” (Peirce 1894 : 6).

As previously acknowledged, the key vehicle for representing mathematical conceptions for Peirce is the diagram. There is a significant passage where Peirce describes the iconic nature of diagrams in mathematics and how they facilitate mathematical reasoning :

The hypothesis of the mathematician is always the conception of a system of relations. In order that they may be reasoned about mathematically, these relations must be conceived as embodied in some kind of objects, but the character of the objects, apart from the relations, is utterly immaterial. They are always made as bare, skeleton-like, or diagrammatic as possible.

Peirce 1895d : 46

From forming a hypothesis, one can see how a semiotic interpretation of mathematical diagrams affects the process of doing mathematics. The significance of the role of diagrams cannot be underestimated : “In the procedure of all mathematics whatsoever, the observation of diagrams plays a great part ....” (Peirce 1903b : 69). In one of the richest passages on mathematics and semiotics, Peirce describes the crucial role of the diagram to developing mathematical ideas :

The mathematician begins his work by making a diagram or scheme. [...] A diagram is a figure whose parts are connected according to a prescription or rule; and any figure whose parts are so connected is as good as any other, except that one is preferable which makes the prescribed connections most prominent, and features not prescribed the least prominent. Allow me to call the mathematician’s diagram or scheme an icon. Then an icon is a mere figure of connected units.

Peirce 1889 : 258-259

Perhaps the last step is to link Peirce’s semiotic conception of mathematics to mathematical economics. In what may be his most mathematically themed monograph in the later years of his life, the “Minute Logic”, Peirce (1902a : 79-80) asserts that the diagram enables a transition from more general qualitative thought to mathematical versions of the sciences including economics. “There will be a mathematical logic just as there is a mathematical physics and a mathematical economics. […] Mathematics is engaged solely in tracing out the consequences of hypotheses [or abductions]” (Peirce 1902a : 112-113).

Near the end of his life, Peirce would provide an interesting interpretation of this semiotic and evolutionary interpretation of mathematics. For Peirce and others, mathematics has very powerful capabilities. What Peirce seems to claim is that the semiotic-relational powers of human inquiry essentially amount to an exceptional human resource. Our ability to explore the world abductively in a forward-looking way with human thoughts taking the form of signs may be humanity’s most evolved cognitive ability : “Our faculty of guessing corresponds to a bird’s musical and aeronautic powers; that is, it is to us, as those are to them, the loftiest of our merely instinctive powers....” (Peirce 1907 : 34). The human ability to represent the external world with semiotic-logical-mathematical symbolizations could be the “loftiest” of our cognitive faculties. Mathematics is a cumulative, semiotically rich evolutionary cognitive process applicable in various ways to nearly every human life process that exhibits some pattern of stable endurance and for Peirce that includes economics.

Peirce’s Mathematical Economics

Among the most diverse interests of C.S. Peirce were economics and especially mathematical economics. However, the economic passages and writings that reference some aspect of economics are few and far between. Economic writings and passages are something like the proverbial needle in the giant haystack of Peirce’s writings. Among Peirce’s philosophical writings, one often finds brief comments and passages from a few sentences to a paragraph or two about economics. At times, he mentions some prominent economists of the 19th century, or he mentions aspects of economic theory like self-interest, or the various properties of goods which economists later call substitutes or complements.

A place to begin is with the only article that Peirce published on economics during his lifetime. Because of its appearance in the Collected Papers, it has been relatively easy to access since the 1950s. It is titled, “The Note on the Theory of the Economy of Research”. The “Note” was part of the Coast Survey documents for 1876, which were published in 1879 (CP 7 : 76-83). The historical proximity of the “Note” to the six “Illustrations” essays (1877-1878 : 109-199) was noted by Max Fisch, who thought Peirce intended to write a seventh economically themed essay based on that piece. Also, appearing with the “Note” in the Collected Papers is a section of a grant application to the Carnegie Institution which further elaborated aspects of the theory of the economy of research (1902b : 84-88). Part of another manuscript commented on the economy of research in relation to the ideas of Ernst Mach (1896 : 48-49).

Peirce’s (1879) “Note,” may be one of the most advanced pieces of mathematical economics of that era (Wible 1994, 2018). In that short article, the claim is made that research in science needs to pay attention to the economic dimensions of scientific accuracy. Peirce created a mathematical model of the net economic benefit of spending more research funds towards increasing the accuracy of scientific measurements. The mathematics of the model is very similar to Stanley Jevons’s (1871) utility-maximizing model for the optimizing consumer deciding about how much to consume of two different foods like corn and beef. However, instead of choices between two foods, Peirce reinterprets the mathematics for the scientist contemplating how to allocate additional funds to two different research projects with the aim of raising the accuracy of each research project. The decision criterion or marginal condition comes down to the additional accuracy generated per additional dollar of expense for each research project. The several lines of mathematical equations for the economy of research represent multiple research projects. They result in a ratio of marginal value or benefit to marginal cost for each research project. For multiple research projects being conducted by the scientist, the marginal condition should be equalized across research projects.

Equations as such come within Peirce’s broad interpretation of “diagrams” and so do graphs representing qualitative and relational features of those equations and the data. Other than the equations, the “Note” was illustrated with a mathematical diagram for the case of two research projects. This two-case diagram closely follows Jevons depiction of marginal utility. Jevons (1871) had provided a bidirectional diagram of the marginal utility model for beef and corn consumption and this graph is quite famous in the history of economics. With regard to scientific projects, Peirce followed the logic of Jevons’ model. He reasoned that the additional accuracy per dollar of additional expense would diminish as more resources were expended on each research project. On a bidirectional graph, the marginal benefit of additional research expenditures would decline both in the usual and in the reverse directions, so that there would be an intersection point somewhere near the middle of the graph. This intersection point was something like a research equilibrium point for a scientist considering how to allocate funds to multiple research projects and attempting to gain the greatest increase in accuracy for the last dollar allocated to each research project.

Peirce’s mathematical and diagrammatic model of the economy of research is interesting in its own terms and reveals that he was probably the most mathematical American economist of that era. But there are a few other writings on the subject of economics that would come to light which reveal a higher level of mathematical reasoning and they appear in Peirce’s New Elements of Mathematics (NEM). Among the textbooks and manuscripts on mathematics and even computation in the New Elements is a brief collection of three writings on economics. One is a letter from Charles to his father Benjamin in December of 1871 (1871a), a second is titled, “Calculus of Wealth” (1871b), and the third, “On Political Economy” (1874). While the letter contains the mathematics of two optimizing firms, it concludes with the tantalizing postscript that “this is all in Cournot”. The other two manuscripts contain mathematical equations for the theory of the firm. They also seem to reveal the influence of Cournot. “Calculus of Wealth” (1871b) and “On Political Economy” (1874) contain many lines of equations for the profit-maximizing firm and the latter reveals several special partial derivatives addressed to some aspects of the behavior of the firm.

Cournot is now famous for being the first to formulate the theory of two firms competing one against the other within the same market. This is the market structure known as duopoly and the optimizing equations in Peirce’s letter to his father are those of the two firms in competition with one another. Among the equations are Cournot’s famous reaction functions in Peirce’s notation. Cournot’s (1838) Researches, was the first monograph to make extensive use of calculus in the formulation of microeconomics. Cournot’s book was the subject of interest of a group of Harvard scientists and faculty members called the Cambridge Scientific Club when they met to consider Cournot’s work in December of 1871. This meeting is of historic interest because it reveals an interest in mathematical economics that was quite rare in North America at that time (Wible and Hoover 2015). Additionally, the mathematical economics of Cournot seems to underpin Peirce’s positions on the Spanish Treaty debate of the late 1880s, which resulted in two letters written to the editor of The Nation. The substance of the letters was about the consequences of placing a tariff on the importation of sugar and about how this might reallocate sugar purchases and production from other nations of the world (Wible and Hoover 2021).

Moving beyond Cournot-style mathematics of the firm, “On Political Economy” comes in two segments and the second qualitative part provides some general remarks about the mathematical logic of the firm and then offers a statement of an axiom of transitivity for consumer choice. Peirce believes this constitutes an important axiom of political economy :

The dependence of demand on price arises from this fundamental proposition. The desire of a person for anything has a quantity of one dimension, and a person having a choice will take that alternative which gives him the greatest satisfaction. In other words if a person prefers A to B and B to C he also prefers A to C. This is the first axiom of Political Economy.

Peirce 1874 : 176

This relational interpretation of consumer choice is truly path-breaking since it reveals a line of thought from the perspective of abstract mathematical logic that would become prominent many decades later. The statement of what economists call the axiom of transitivity would come to the attention of economists in the 1950s. That Peirce was conceiving of consumer theory in terms of abstract mathematical properties in the mid-1870s is truly extraordinary. In this regard, an abstract mathematical interpretation of the logic of consumer preference would certainly be more sophisticated than Jevons’s interpretation. Furthermore, it should not be forgotten that Peirce already had begun to conceive of mathematics in semiotic terms about seven years earlier.

Another major episode of the importance of economics in Peirce’s writings comes in the Harvard Lectures (1903a). In the first lecture, Peirce provides three examples of the logic of pragmatism. One of them involves games of chance and the other how to reason when the repetition of moves in the games gets quite large. The third is the optimizing calculus of the profit maximizing insurance firm with the probability of loss appearing in the model as a mathematical parameter. There Peirce actually provides about ten lines or so of mathematical derivation of the optimizing conditions including adjustment toward the equilibrium level of contracts from both above and below the equilibrium point (Wible 2014; Hoover and Wible 2020).

As it turns out, from the early 1880s forward, Peirce’s interest in mathematical logic had intersected with Cantor’s theory of infinite sets. Cantor’s theory involves counting the number of members of a collection and deciding whether that count is large but finite or perhaps even infinite. If a collection is thought to be infinite, one could imagine whether the collection is generated by a mathematical process with a simple equation or operator or whether the set is infinite but without a defining operator. For those infinite sets without a specific mathematical operator, he imagined placing the members in some relational order. Thus, the logical property of transitivity becomes of paramount importance. A third aspect of the logic of infinite sets was providing a procedure which would add one set of infinite points to another set of infinite points.

Peirce’s efforts to provide an argument for the meaning of mathematical concepts and processes which involve infinity and continuity also found expression with regard to economics. The economist who most interested him was David Ricardo. It was Ricardo’s theory of rent, which provided the possibility of a real world application of a conception of calculus and eventually infinite sets. Writing before the creation of set theory, Peirce used the term collection. Peirce claims at one point that “the reasoning of Ricardo in his theory of rent, reasoning which is of fundamental importance to political economy, as well as much of the elementary reasoning of the differential calculus, is of that nature. But these are only exceptions which prove the rule” (Peirce 1897 : 176). At another point he refers to “The peculiar reasoning of political economy; the Ricardian inference” (1890 : 21). But what is Ricardian inference? Peirce had repeatedly used a really well-known example for illustrating the problem of filling in the gaps between the points of an infinite collection. That example was Achilles and the tortoise. As intuitively framed, Achilles the runner starts behind the tortoise and, of course, he runs much faster than the tortoise crawls, and he closes the gap between them by a factor of half over each interval of time. One can understand that closing the gap by fifty percent each time implies that Achilles would never catch the tortoise. However, one could put the rates of running and crawling into mathematical form and one could solve for the point when Achilles would catch the tortoise. This is the Achilles mathematical example that Peirce repeatedly discussed with William James. Apparently, James would never accept that Achilles would catch the tortoise or that it was meaningful to think of infinity and continuity in this way.

While Peirce did not include the algebra of infinite collections in the Harvard Lectures, he had created a concept of reasoning related to those collections, which he called Ricardian inference as noted above (Hoover and Wible 2020). Ricardo in his theory of rent had used the logic of sequential, marginal adjustment for the farmer contemplating bringing additional plots of land into cultivation. For Peirce, this could involve the logic of an infinite collection where the members could be relationally ordered but not with a generating function. With various amounts of rain, fertilizer, time, and methods of cultivation, the number of possible productivity options for the landowner’s plots in conception could be infinite. This logic of infinite collections and sets is really, what he wanted to present in the Harvard Lectures, but instead he substituted the calculus of the profit maximizing insurance firm. The insurance firm, like Ricardo’s landowner contemplating marginal productivity and rent, essentially conceived of its economic problems in several logical and mathematically equivalent ways : as a large collection of phenomena, as involving the logic of infinite collections, and also as involving the marginal decision conditions from the calculus of marginal productivity theory.

Turning back more generally to Peirce’s semiotics, mathematics, and philosophy - one of Peirce’s diagrams can be used to illustrate important strands of topological like connections among the various strands and branches of his contributions. One of his important arguments is that philosophical problems of the human mind as they were typically taken up by philosophers and psychologists could be analogously conceived with mathematics and logic. The problems of mathematics and logic could be externalized so that unpsychological processes of reason and criticism could be inter-subjective and objective. Something similar could happen with the sciences. Diagrams could be used as an inter-subjective vehicle for reasoning about the patterns of processes that were thought to be in characteristic of the real processes being investigated by that science or the mathematician. Similarly, what is possible diagrammatically for representing reasoning processes in philosophy, mathematics, and the hierarchy of the sciences might also be done with Peirce’s own contributions.

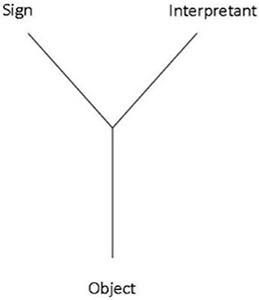
Of course, one way to summarize all of this would be with a diagram or two closely related to one of Peirce’s diagrams. Across many of his intellectual contributions, Peirce emphasized triadic connections and relations. One of his favorite representations of a triadic relation was a diagram with three lines where one end of each line meets in the middle like the spokes on a wheel. This is what might be called a “three-spoke” diagram even though it was identified literally as a “furcation” in his topological writings. Peirce’s triadic diagram, the three-spoke diagram, can be found in Figure 1. Sometimes it has the single end up; at other times, it is down. While Peirce emphasized repeatedly the inherent and unbroken unity of relational triadic connection and brought that understanding to his semiotics, he never combined the two in a diagram like Figure 1. However, I believe this can be done by assigning the three aspects of semiosis to the three-spoke diagram at the outward end of each spoke – sign, object, and interpretant. The sign is an external representation of an external object or process and the interpretant is the corresponding internal interpretative representation in the mind of the inquirer. The interpretant is a cognitively constructed understanding of the relation between the sign and its object and in that way is connected to both. All three aspects of semiosis are essential or the sign degenerates and ceases to indicate meaning to the interpreter. Figure 1 itself is a diagrammatic sign of the triadic interrelatedness of each aspect of the sign.

Fig. 1

The Triadic Relational Nature of Peirce's Semiotics

The Triadic Relational Nature of Peirce's Semiotics

-> Voir la liste des figures

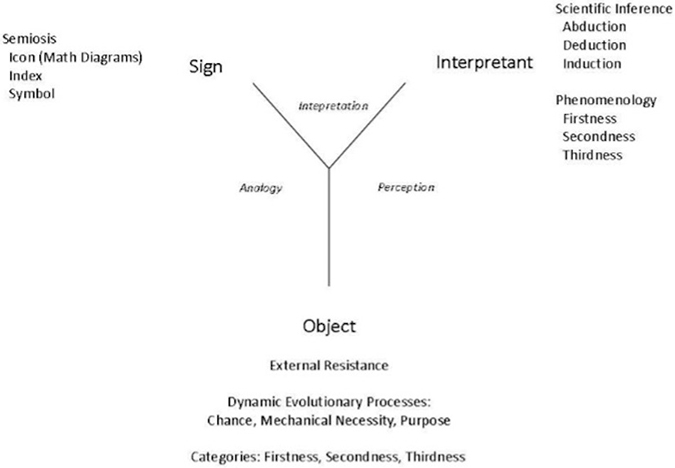
Each corner of the triadic diagram may be further triadic in an important way. A second graph, Figure 2, adds major important strands of Peirce’s philosophical outlook and writings to the triadic figure from the first diagram. Many of these strands are substantive and significant in their own right and they would require much more elaboration than is possible in this short essay. But Figure 2 indicates how many of the most important strands of Peirce’s contributions might be interrelated. Starting with the upper left spoke, denoted as “sign,” signs of course have three initial broad categories : icon, index, and symbol and each of those may have further specialized versions. There are many more kinds of signs, which have been explored by subsequent semioticians. Additionally, there are the many specialized signs of mathematical inquiry and logical demonstration as noted previously. Turning to the upper right spoke, the interpretant is the systematically constructed understanding by the human interpreter and it involves taking that inquirer through the recursive loops of the reasoning processes of abduction, deduction, and induction in order to create, criticize, and correct any problems with the representation or observation of external phenomena with signs. Also placed here is Peirce’s phenomenology of human thought in terms of three relational categories alongside the processes of reasoning : abduction, deduction, and induction. More on the relational categories can be found with the lower spoke.[6]

Fig. 2

Further Triads of Peirce's Semiotic Conception of Relation Human Understanding and Scientific Explanation

Further Triads of Peirce's Semiotic Conception of Relation Human Understanding and Scientific Explanation

-> Voir la liste des figures

At the end of the lower spoke termed the “object” are Peirce’s three metaphysical categories, which focus on the relativity simplicity or complexity of relational patterns in the objects out there and external to the human mind. Some patterns of objects are monistic or homogenous in character, others are dualistic, and then some evolve into triadic complexity. Peirce gave his categories of external phenomena the mathematically referenced names of Firstness, Secondness, and Thirdness. Peirce thought of evolution as a recursive process where entities would move from processes of firstness to secondness, from secondness to thirdness, and even back to secondness. Broadly speaking, firstness is the category of origin and possibility; secondness is the category of dual relationships like matter, constraint, equilibrium and death; while thirdness is the category of unfolding and living complex events and processes like mind and active mental processes. The categories also need to be capable of being internally represented and apprehended in the mind, which led Peirce to a phenomenology in terms of the categories as well. Peirce also commented more broadly on evolutionary processes as unfolding in three basic ways : chance, mechanism, and higher purpose. These are represented on this lower spoke as well. The three spoke diagrams of Figures 1 and 2 are representations of the abstract interrelatedness of Peirce’s writings and philosophy and they are topological-semiotic figures in their own right. Those diagrams can also illustrate how the major strands of Peirce’s major contributions and writings might fit together as well. As such, a topological representation seems perfectly fitting to portray the stranded and labyrinth style of Peirce’s system of ideas. The purpose of Figure 2 is to illustrate the triadic complexity of Peirce’s thought and to see how his theory of signs combines external objects and processes with semiotic representations of those events and processes. Furthermore, they portray how meaning may be constructed by the human interpreter using signs to make some reliable sense of our world. Economics is no exception to this semiotically conceived topological process of how humans have the possibility for understanding how the natural and social worlds function, how they can meaningfully communicate about those worlds, and how anyone could know anything about an uncertain and evolving world and economy in a reliable way.

Herrmann-Pillath’s Foundations of Economic Evolution and Peirce’s Semiotic Economics

Given Peirce’s deep interests in the political economy of his own time and the most advanced mathematical economics of the late 19th century, one might imagine that those interests would be well-known within economics and that there would be significant interest in his pragmaticist or mathematical-logical version of pragmatism and scientific method. However, that is not the case. It is very likely that the preceding succession of narratives from pragmatism, to semiotics, to mathematical semiotics, to mathematical economics appear here for the first time. Themes like the multi-stranded, topological nature of Peirce’s intellectual style, his path-breaking contributions to semiotics, his unique semiotic interpretation of mathematics, and finally his interests in classical economics and neoclassical mathematical economics likely have not been connected in this manner previously. Peirce is mostly unknown in contemporary mainstream economics although there is growing interest among a few across several generations of scholars. Outside of mainstream economics, Peirce is somewhat better known. The once dominant school of American institutionalists often claim Peirce as a founding influence but, like mainstream economists, they seem to be totally unware of the mathematical-semiotic thread of his evolutionary philosophy, pragmaticism, and mathematical economics (Wible 2015).

American institutionalists view themselves as evolutionary economists. This is in contrast to the mainstream, which they view as too mechanistic and often as too mathematical. One can get an idea of their views on mathematical economics from Thorstein Veblen who was a famous critic and contributed the name of “neoclassical” economics. Veblen (1898; 1919) in several essays on the nature of economic science, claimed that the emerging mathematical theory of marginal utility in late 19th century economics was being applied in a mechanistic way. He viewed economists functioning more as engineers rather than as scientists inquiring about deep theoretical reasons for broad patterns of human behavior. Veblen thought that the unit of analysis should be the institution rather than the individual. He was particularly famous for creating two categories of analysis for the consumer or the firm where one evolutionary category of analysis was held to be closer to the intuitions that humans have in the flow of social and economic processes. The other category was mechanistic and involved the imposition of quantitative counting, measuring, or theorizing in some important way thus distorting the sense of evolutionary flow and social connection. His pecuniary, emulation, and finance motives were mechanistic conceptual constructions encapsulating the economic analysis of his time, which, in his view, led to significant distortions of conceptions of economic activity.

What is interesting about Veblen is that he actually took a class from Peirce during the academic year he spent at Johns Hopkins University in 1881 (Houser 1986 : li). He enrolled in Peirce’s elementary logic class. About two decades later, at the same time when Veblen was increasingly criticizing the rise of neoclassical mathematical economics, Peirce was giving lectures on the role of mathematics in facilitating an understanding of the world. The first of Veblen’s several articles was his “Why is Economics Not an Evolutionary Science?” (1898) published by the Harvard economics department in its new journal, the Quarterly Journal of Economics. During that same year, Peirce (1898) delivered his Cambridge Conference Lectures specifically titled, “Reasoning and the Logic of Things”. For this set of lectures, Ketner and Putnam (1992) proposed the title “The Consequences of Mathematics”. Peirce and Veblen, professor and graduate student, seem to offer almost entirely dissimilar visions of the place of mathematics in understanding the world and the economy. This is important, for it is often claimed that Veblen learned his evolutionary vision of the world from Peirce. If so, the mathematical piece is a problem. A fuller exploration will soon appear on this very subject (Wible 2021). Until recently, the institutionalist, who seems to have had the most to say about Peirce, was J. R. Commons (1934), in his Institutional Economics. Commons presents Peirce as the most important philosopher since David Hume.

While many evolutionary institutionalist economists have claimed Peirce in one way or another as a founding level influence, the one who has done it most expansively is Carsten Herrmann-Pillath. Herrmann-Pillath has authored what is by modern standards a massive work, Foundations of Economic Evolution : A Treatise on the Natural Philosophy of Economics (2013). Several things strike one as very Peircean in Herrmann-Pillath’s treatise. Obviously, the author provides an interpretation of Peirce’s semiotics with innovative extensions, such as links to conceptions of causality from Aristotle such as formal, final, and efficient causality (7). Second, Herrmann-Pillath makes extensive use of diagrams with many of them being variations of his diagrammatic presentation of semiotics. The only person whose work has greater diagrammatic density or more illustrations per hundred pages of publication is probably Peirce himself. Third, there are many mathematical equations, and several logical expressions that are treated as equations. Fourth, there are several market or supply-and-demand diagrams in the last chapter on markets, which clearly have mathematical counterparts.

Consider Herrmann-Pillath’s exposition of Peirce’s semiotics. In the first chapter of his work, Herrmann-Pillath maintains that a natural philosophy of economics needs some reference to an external material world. There, Herrmann-Pillath adapts Peirce’s semiotic framework to achieve that externalizing role. Peirce’s semiotics aims at making a connection with the external world, which is why it forms such an important role in Herrmann-Pillath’s Economic Evolution. As an evolutionary indeterminist, Peirce considered the world encountered by scientists and humans external, without the solidity or firmness implied in mechanistic or materialistic conceptions of the natural and social worlds :

The only end of science, as such, is to learn the lesson that the universe has to teach it. […] But insofar as it does this, the solid ground of fact fails it. It feels from the moment that its position is only provisional. It must then find confirmations, they are only partial. It still is not standing upon the bedrock of fact. It is walking upon a bog, and can only say this ground seems to hold for the present. Here I will stay till it begins to give way. Moreover, in all its progress, science vaguely feels that it is only learning a lesson.

Peirce 1898 : 176-177

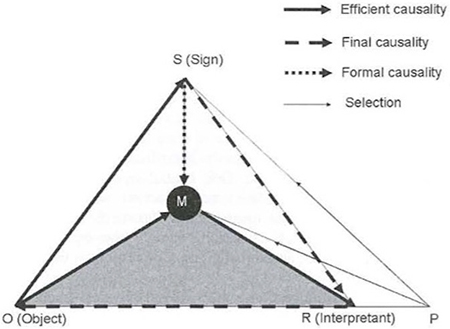
Many if not most of those lessons take a semiotic and mathematical form. In the second diagram of Economic Evolution (23), the Peircean semiotic triad of sign, object, and interpretant is presented in slightly altered form as sign, object, and observer. There the three aspects of a theory of signs correspond with the three points on the corners of a triangle. However, this triangular presentation of Peirce’s semiotics quickly gives way to a much richer triadic diagram near to the end of the first chapter. To my knowledge, Peirce never used the triangle to represent triadic relational complexity. Instead, another graph from his writings was adapted for that purpose, the three-spoke diagram as displayed previously in Figures 1 and 2.[7] The reason for this is that a triangle could imply that the fundamental pattern of connection among the concepts at the corners is fundamentally dualistic or two at a time. Peirce’s interpretation of triadic relation implies that the fundamental significance of thinking in signs inheres in the simultaneous conjoint complexity of its three different aspects in indecomposable unity. As Houser has stated in the quote above, if one of the aspects of a sign is taken away, the whole sign relation collapses.

However, Herrmann-Pillath moves rather quickly to a diagram that comes quite close to resembling Peirce’s three-spoke triadic diagram. In the eighth figure of Economic Evolution (41), Herrmann-Pillath places an M in the middle of his semiotic triangle and connects it with the points at the corners of his semiotic triangle : sign, object, and observer as shown in Figure 3. M seems to stand for a connecting mechanism or relation between the three modes of semiosis. With the M in the middle and connected to the three points on the corner, this semiotic triangle gains the three-spoke character of Peirce’s analysis of undegenerated triadic relatedness. The lines of the triangle are still left in place and are designated as offering other cognitive features of semiosis. After this eighth figure of chapter one, nearly every subsequent semiotic diagram in Economic Evolution has the middle point of triadic relational unity which is crucially important in making the analysis truly Peircean. If all of the semiotic M-centered and triadic semiotic illustrations are considered in relation to one another, then they and their interpretations form the theoretical back-bone of Herrmann-Pillath’s evolutionary theory. Of course the similarity of Herrmann-Pillath’s three-spoked diagrams to Peirce’s does not make Herrmann-Pillath’s approach Peircean as such. Peirce mostly wrote about semiosis in words with his use of diagrams mainly in his writings about mathematics and the sciences. Instead, Herrmann-Pillath’s adoption of Peirce’s theory of signs coupled with his separate and unique penchant for representing that theory diagrammatically may account for much of the similarity between his and Peirce’s diagrams. Also, there is no doubt a topologically limited number of ways to diagram triadic relationships.

Fig. 3

Hermann-Pillath's M-Centered Triadic Semiosis Diagram p.41

Hermann-Pillath's M-Centered Triadic Semiosis Diagram p.41

-> Voir la liste des figures

The significance of Herrmann-Pillath’s M-centered triadic diagram does not end here. Indeed, there is in fact a geometric illustration in Peirce’s writings very similar to that diagram but it is found in his drafts of mathematical textbooks rather than in the writings on semiotics. Herrmann-Pillath, like so many others who have been interested in Peirce, seems to look past his writings on mathematics as found in New Elements of Mathematics (1976). As mentioned above, in the latter part of the 19th century, Peirce became very interested in a new branch of mathematics that would become topology. He became deeply intrigued with the abstract algebraic properties of many different sorts of geometric diagrams, lines, spaces, surfaces, solids, and knots and the logical relations exemplified therein. He went so far as to include draft sections on topical geometry in those mathematical texts (1895a, 1895b). At one point, he was developing the algebra and logic of closed surfaces and solids and he provided a triangular illustration shown here in Figure 4 (1895a : 190). Figure 4 is from a draft of the second Harvard Lecture (1903c : 155) where Peirce was explaining the logic of mathematics. It was intended as a diagram of the possible relations of four different points. This could have been some of the more explicit mathematical themes that James asked Peirce not to present in the Harvard Lectures, which seemed to lead him to substitute the mathematics of the insurance firm. It may have a touch of irony but note how similar Peirce’s graph in Figure 4 is in appearance to Herrmann-Pillath’s M-centered diagram in Figure 3. In total, a careful count reveals 29 of these M-centered semiosis diagrams, which form the main theoretical framework of Economic Evolution. While Peirce and Herrmann-Pillath make different applications of their diagrams, the similar appearance of their figures suggests that Herrmann-Pillath’s geometric diagrams have a rich topological nature as well. In terms of diagrams, it is this shared topological similarity with Peirce, not directly acknowledged by Herrmann-Pillath, which suggests an important intellectual similarity between Peirce’s writings and Herrmann-Pillath’s treatise. Also, the scaffolding of 29 triadic semiotic diagrams in the treatise and their portrayal of triadic relationships occurring across many social, economic, and natural phenomena which are linked to Peirce’s conception of semiosis, make this one of the most Peircean works in the history of economics.

Fig. 4

From Peirce's "Lectures on Pragmaism, Lecture II (draft) NEM IV, 1903 p.155

From Peirce's "Lectures on Pragmaism, Lecture II (draft) NEM IV, 1903 p.155

-> Voir la liste des figures

Besides the diagrammatic representations of semiosis, other entities also function as diagrams, according to Peirce. His conception of diagrams includes not only diagrams with implied relational structures; it also includes any structured array or presentation of numbers, letters, symbols, lines, and arrays of those items with labels and designations. In contrast to most works from an evolutionary perspective in economics, there are many equations in Economic Evolution. A relatively careful but quick count reveals 91 equations and logical expressions set off on separate lines, as they would be in a presentation by a mathematical economist. Those equations are about the specific patterns and processes of thermodynamics, entropy, randomness, biology, information, and other evolutionary processes. The accompanying triadic, M–centered and corner-expanding diagrams are intertwined with interpretations of the equations. In the last chapter on “Markets”, several other market diagrams certainly have well-known systems of equations from mathematical economics behind them.

Other than the detailed equations of mathematics and the intricate symbolic statements of abstract logic, the purpose of diagrams is to look beyond the intricate details and represent the fundamental patterns of relation among constituent entities in a process or collections of processes as clearly as possible. To perform their role as analogs of what could be more complicated and intricate patterns of relationships, diagrams would need to be constructed following widely agreed upon conventions. If the rules were well understood and the diagrams creatively constructed, then they could facilitate scientific investigation and research proceeding more economically and efficiently. Peirce seemed to believe that diagrammatic and mathematically facilitated science including economics would lead to faster if not greater scientific discovery. The sheer multiplicity of diagrams in Foundations of Economic Evolution suggests that many more economic processes could be approached in a relational, mathematical, and semiotic way. Many of Herrmann-Pillath’s diagrams are quite novel and unconventional and so is an awareness of Peirce’s semiotic interpretation of mathematics and of evolutionary processes. It may thus take a considerable amount of time for the full importance of Foundations of Economic Evolution to be realized in economic and other social science research.

There is a loose end or two that deserve further comment. One is that Herrmann-Pillath says very little in Economic Evolution about the traditional conceptions of the individual in economic processes or households and firms. Like Veblen, Herrmann-Pillath mostly focuses more at an aggregative level. It is clear that while Veblen wanted to take economics in a different direction towards an evolutionary perspective, he did align his categories of analysis with those of neoclassical economics. Herrmann-Pillath provides alternative conceptions of consumption, business activity, and social processes at higher levels of aggregation than the optimizing theories of neoclassical economics. His Economic Evolution is such a long treatise that one should not expect it to include every social process at every level of analysis. Indeed Veblen wrote several monographs on consumption and the business enterprise. Similarly, the theoretical framework of Economic Evolution could be extended to the firm and even to the household in a more focused way. Actually, Herrmann-Pillath and a co-author seem to have begun to do so. In Macedo and Herrmann-Pillath (2019), the theoretical framework of Economic Evolution is extended to the corporation. The triadic-semiotic theoretical structure of the M-centered and spoke-like diagram is taken and then adapted to the natural and social environments of the corporation. These expansions suggest hierarchies of conception and representation tracking the patterns of roles as the corporation evolves, with ever expanding complex functions in society.

The extension and application of the evolutionary semiotic framework to the corporation makes no mention of the neoclassical theory of the firm, especially in its mathematical version. This sets up an important contrast between Peirce and Herrmann-Pillath. When most of Peirce’s intellectual contributions are taken together, it is clear that his neoclassical mathematical examples need to be interpreted as illustrations of his semiotic conception of mathematics and pragmatism. The optimizing calculus of utility and profit maximization were meant as illustrations of the logic of economic activity and the logic of large collections of phenomena relating to the mathematical questions of continuity and infinity inherent in economic phenomena. They were not meant to imply an adoption of the utility theory of individual behavior or anything like a substantial acceptance of British empiricism and utilitarianism that emanated from the classical economics of James and John Stuart Mill or other British economists of the 19th century.

All this may mean that Peirce’s and Herrmann-Pillath’s evolutionary semiotic conceptions of scientific and economic processes may have significant complementarities. It is likely that Peirce would have recognized that there are larger scale and more aggregative patterns of relationships in economic activity than those in the individual patterns of conventional economic theories of the consumer and the firm. Peirce’s interest in what is now regarded as neoclassical mathematical microeconomics should not be taken to mean that he adopted the neoclassical, utilitarian view of economics. Instead, he was creating a conception of the individual in the processes of living human inquiry as being embedded in semiotic, relational, and somewhat mathematical processes of cognition. A similar semiotic conception of the individual in evolutionary processes is also found in Herrmann-Pillath’s Economic Evolution, where it is focused more at higher levels of aggregation. Again, this suggests a complementarity of perspective between Peirce and Herrmann-Pillath. Much can be learned by juxtaposing the myriad works of C. S. Peirce with those of Herrmann-Pillath. The comparison might bridge some of the more significant intellectual and scientific differences between various schools of contemporary economics.